| ENG RUS | Timus Online Judge |
1360. Philosophical DisputeTime limit: 2.0 second Memory limit: 64 MB One day, mathematician and philosopher were engaged in a heated dispute. Philosopher said: — Ideal line has only length and no width, therefore, no line can have an area. Mathematician replied: — That's as it may be, but still you can fill a square with a line in such a way that there will be no gaps. And you can't deny that a square has an area, and he grinned. But Philosopher still wasn't convinced: — Show me this line, then. — With pleasure… — responded Mathematician and scribbled some equations on a piece of paper: 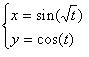 — With t increasing, the point (x, y) will move around the square, forming a line. — So what? — asked Philosopher. How is it going to fill the entire square? — Indeed, it will, — said Mathematician, — Whichever point inside the square you draw, the line will eventually cross that point. — No, — replied Philosopher indignantly, — Anyway, I don't believe. When will the line cross this point? — and he put a thick dot inside the square. Give Philosopher an answer. InputThe first line of input contains the coordinates (x0, y0) of the dot center (−1 ≤ x0, y0 ≤ 1). The second
line contains ε ≥ 0.0001 — the radius of the dot (the dot is essentially a small circle). OutputAny value of t in the segment [0, 1012], which corresponds to the line crossing the dot, or "FAIL", if the line doesn't cross the dot. Sample
Problem Author: Stanislav Vasilyev (idea by Den Raskovalov) Problem Source: IX Collegiate Students Urals Programming Contest. Yekaterinburg, April 19-24, 2005 Tags: mathematics |
